
Shashank Jaiswal
I'm a software developer with expertise in distributed systems. I'm a graduate of Purdue University [PhD (Aeronautics), double MS (Physics, Aeronautics)], and Indian Institute of Technology [B.Tech (Mech)]
Effect of wall curvature on thermally driven microchannel flows
Shashank Jaiswal
2025 American Institute of Physics (AIP), Physics of Fluids, Accepted: 1 April 2025
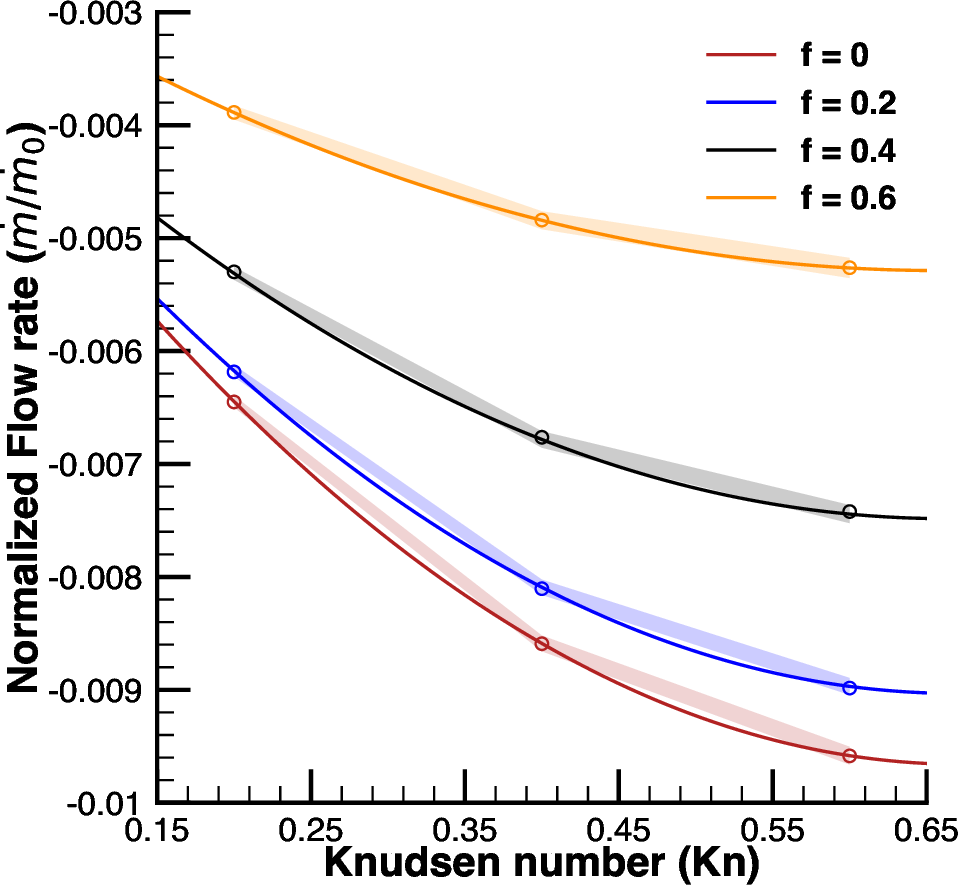
Abstract: Low-speed flows in microscale geometries are of fundamental importance in microelectromechanical systems (MEMSs), including temperature-driven Knudsen micropumps (KPs). Microchannels are the key elements of KPs that ensure the gases are in a rarefied state. The geometric structures of these microchannels consist mainly of plates and ratchets. In this work, we systematically explore the design of these microchannels with curved walls. More precisely, we introduce a perturbation on the top wall of the microchannel using a smooth infinitely differentiable cosine damping function, which allows us to understand the effect of small changes in curvature on the system response. With a linear increase in perturbation, the diameter of the microchannel decreases smoothly toward the center of the microchannel located at x=1.25 μm. The magnitude of the mass flow rate calculated along the vertical line x=1.25 μm decreases quadratically with increasing perturbation. Along the horizontal line y=0, the flow speed follows a Gaussian-like profile with increasing perturbation. Additionally, this Gaussian-like profile is not symmetric with respect to the vertical centerline x=1.25 μm. Over the surface of the curved wall, the flow speed follows a bimodal behavior with increase in curvature. These observations also hold at different Knudsen numbers in the transition regime. Several other flow properties of interest, e.g., density and temperature, have been reported. These observations follow from kinetic theory simulations of the Boltzmann equation carried out using the discontinuous Galerkin fast spectral method, which produces noise-free solutions for low-speed flows compared to the traditional direct simulation Monte Carlo method.
An entropy stable scheme for the non-linear Boltzmann equation
Shashank Jaiswal
2022 Elsevier, Journal of Computational Physics, Submitted: 9th Nov 2021, Accepted: 6 May 2022
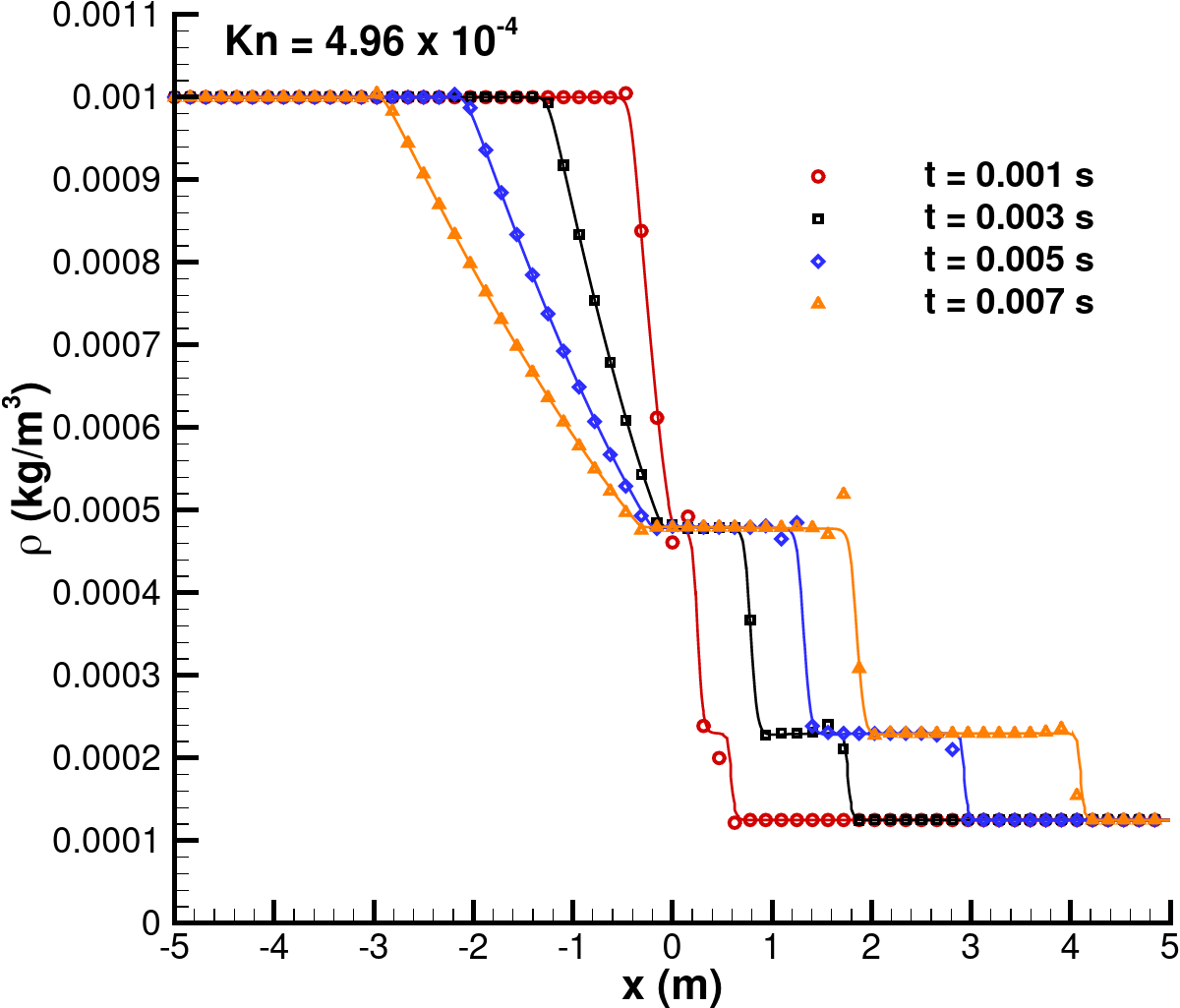
Abstract: The kinetic equations govern the behavior of gaseous flows, compressibility, turbulence, reactions with internal energy exchange, that form the essence of numerous physical processes. Despite their wide applicability, their six-dimensional (seven including time) nature presents a huge computational challenge. With the advent of the modern high performance computing systems and few recent advances in numerical methods, it is now possible to numerically study the behavior of these systems. However, to understand the instability of rich molecular processes and find a structure in the apparent chaos, a scheme that is efficient in multi-dimensions, exhibits high parallel-efficiency, and foremost produces an entropy solution as per the theory of solutions of hyperbolic systems may prove useful. In this work, first, we construct an entropy stable flux for non-linear inhomogeneous (full) Boltzmann equation. Second, to ensure geometrical flexibility, we couple the scheme with a class of high order discontinuous Galerkin discretization which satisfy summation-by-part (SBP) discretely. Third, utilizing SBP, we prove that the resulting semi-discrete scheme is locally and globally conservative in flat spaces; efficient and simple; and therefore suitable for dealing with highly complex non-smooth flow problems. Fourth, we show that the fully-discrete kinetic scheme, utilizing an implicit-explicit time-discretization, in the limit of vanishing Knudsen number, becomes an entropy-stable explicit scheme applied to the Euler system. Fifth, we carry out a series of verification tests to illustrate the stability, accuracy, and conservation properties of the proposed method. These tests involve over 85 million degrees of freedom and over 50 billion operations per time-step.
Third research monograph written during PhD ... probably the only student at Purdue to pull it off!The intent of PhD is to produce independent researchers. So, I thought let's write a single-author paper. So, I wrote a paper and submitted it for review to CMAME. Then I thought what if the paper gets rejected, so I wrote the second paper and submitted it to JCP. Then I thought what if the second paper gets rejected, so I wrote the third paper.
To prospective graduates: You need a certain level of intelligence. Beyond that it's a matter of discipline and desire to excel.

Non-linear Boltzmann equation on hybrid-unstructured non-conforming multi-domains
Shashank Jaiswal
2021 Elsevier, Journal of Computational Physics, Submitted: 16th Jan 2021, Accepted: 2 September 2021
Abstract: Adaptivity is crucial for addressing practical challenges of the next decades. In this regard, recent surveys highlight that it continues to be a major bottleneck in computational fluid dynamics workflow. We introduce mixed non-conforming discontinuous Galerkin discretization for the full Boltzmann equation in 2D/3D. These schemes have been designed for efficiency - motivated in part by spectral and isogeometric weighted collocation methods - and retain an optimal O(p + 1) convergence for a p order approximation for non-linear kinetic systems on non-orthogonal grids. In this setting, it is possible to analyze highly complex problems of industrial strength i.e., structured, unstructured, mixed, irregular, multi-domain (multi-block) adaptive geometries at massively parallel scale (ten thousand cores or beyond). Mixed domains permit flexible mesh generation, whereas local nature of discontinuous Galerkin permits construction of adaptive numerical schemes that scale well. To address flows in mixing regime (low/high rarefaction), we couple the scheme with an asymptotic preserving implicit-explicit time discretization. These schemes are iteration free and applicable for a wide range of rarefied flows from free-molecular to continuum. To ensure stability in presence of shocks, we describe a method of constructing limiters on non-conforming grids. Finally, we show that the computational overhead for solving kinetic equations on non-conforming structured/unstructured domains is negligible relative to conforming domains. So, there is no reason to not prefer non-conforming unstructured domains.
Second monograph written during PhD ... probably the only student at Purdue to pull it off!To prospective graduates: Do not let your persistence defeat you.
Isogeometric schemes in rarefied gas dynamics contexts
Shashank Jaiswal
2021 Elsevier, Computer Methods in Applied Mechanics and Engineering, Submitted: 31st May 2021, Accepted: 10 May 2021
Abstract: Geometrical complexity is typical of missile and reentry systems, small-scale devices with intrinsic milli-to-micro scale features, or in general topology-rich systems. Additionally, at high altitudes or at microscales, when the flow becomes rarefied, the conventional continuum/macroscopic equations produce unsatisfactory results. A microscopic/kinetic description becomes necessary. In this work, we introduce isogeometric (IG) kinetic schemes (KS) for the full Boltzmann equation and related kinetic models (Bhatnagar–Gross–Krook (BGK), Ellipsoidal statistical BGK, and Shakov). These schemes are specifically aimed for modeling flows in topologically complex "multi-patch" geometries such as those constructed using computer-aided geometric design tools; high order accurate; unconditionally time-stable (as per our tests) following generalized-alpha method; non-linearly stable following streamlined upwind Petrov–Galerkin method applied at every patch level with patch-to-patch coupling enforced either strongly by constraining the interface using single degree of freedom, or weakly using numerical fluxes as in discontinuous Galerkin (useful because the underlying system is first order in space); amenable to nearly-linear parallel efficiency owing to the six-dimensional nature of Boltzmann equation, wherein the convection and collision can be, respectively, performed simultaneously over velocity and physical spaces. In particular, since the surface representation is exact in IG, when surface properties are to be computed, which is often the case in aerodynamic applications, these schemes perform well. A series of verification tests for 1D/2D/3D-3V initial–boundary value and boundary-value flow problems, involving momentum, energy, and diffusive transport, including problems posed on non-conforming domains/patches, is performed to illustrate the stability and accuracy of the proposed method.
First monograph written even before PhD preliminary examination ... probably the only student at Purdue to pull it off!To prospective graduates: Independence instills the courage to live life on your own terms.
2nd Masters:
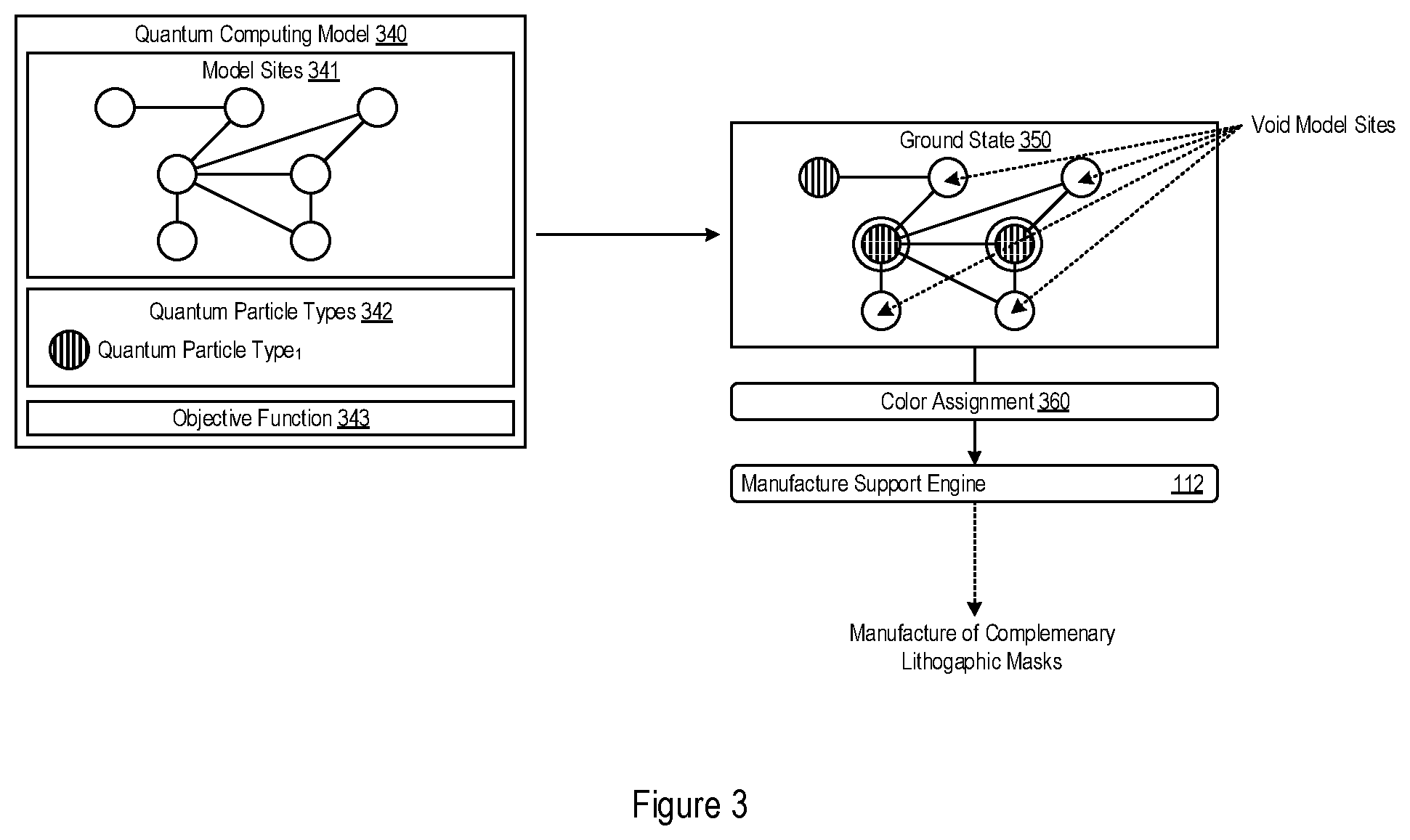
Limited basis quantum particle definitions in applications of quantum computing to electronic design automation processes
Shashank Jaiswal
2020 United States Patent, Filed: 19th Nov 2019, Granted: 24th Nov 2020
Abstract: A system may include a quantum model engine configured to generate (e.g., load or instantiate) a quantum computing model to represent an electronic design automation (EDA) process for a circuit design. The EDA process may be a multi-patterning process to assign colors to geometric elements of the circuit design. The quantum computing model may include quantum particle types that may be defined to prohibit non-physical states in the quantum computing model from occurring. The quantum model engine may also be configured to generate a color assignment for the geometric elements of the circuit design through the quantum computing model. The system may also include a manufacture support engine configured to use the color assignment to support manufacture of circuit layers of the circuit design through multiple manufacturing steps.
My first patent in quantum computation!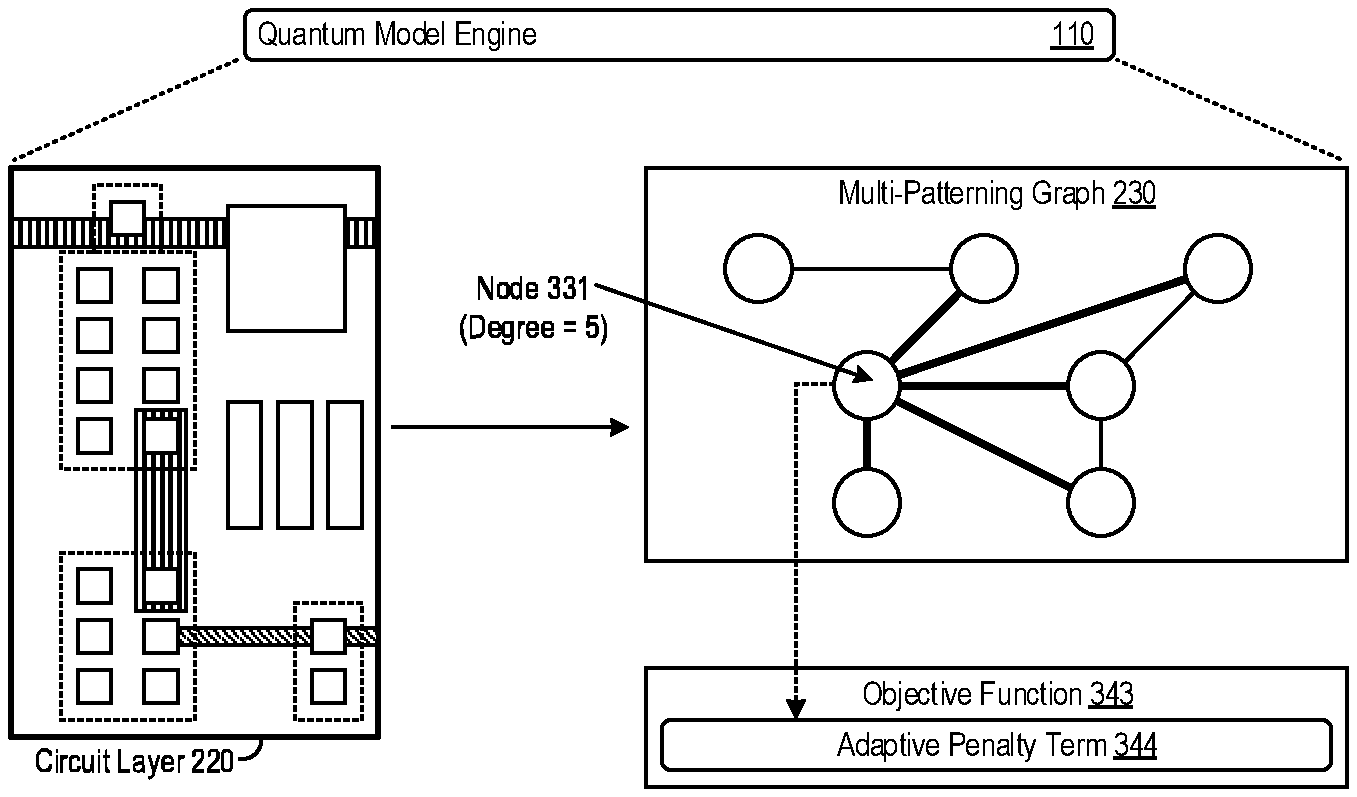
Adaptive penalty term determinations in applications of quantum computing to electronic design automation processes
Shashank Jaiswal
2020 United States Patent Application, Filed: 19th Nov 2019, Assigned: 9th July 2021, Granted: Pending
Abstract: A system may include a quantum model engine configured to generate (e.g., load or instantiate) a quantum computing model to represent an electronic design automation (EDA) process for a circuit design. The EDA process may be a multi-patterning process to assign colors to geometric elements of the circuit design. The quantum computing model may include quantum particle types that may be defined to prohibit non-physical states in the quantum computing model from occurring. The quantum model engine may also be configured to generate a color assignment for the geometric elements of the circuit design through the quantum computing model. The system may also include a manufacture support engine configured to use the color assignment to support manufacture of circuit layers of the circuit design through multiple manufacturing steps.
Second patent (filed) in quantum computationMasters/PhD:

CDS&E: DEterministic Evaluation of Kinetic Boltzmann equation with Spectral H/p/v Accuracy (DEEKSHA)
Shashank Jaiswal, Jingwei Hu, and Alina A. Alexeenko
Award Abstract #1854829, 2019 National Science Foundation, Computational and Data-Enabled Science and Engineering (CDS&E)
Abstract: The goal of this project is to develop an open-source massively parallel computational software DEEKSHA (DEterministic Evaluation of Kinetic Boltzmann equation with Spectral h/p/v adaptivity and Accuracy) for scientific simulation of rarefied gas flows. Such gas flows occur in microdevices or at low gas densities, as in high-altitude atmospheric phenomena and in manufacturing processes that require vacuum conditions. Verification benchmarks and end-user demos will be developed to facilitate adoption of the new scientific simulation software by academia and industry and to provide the user and contributor base for sustained development. Specifically, the demonstration cases will include several classical rarefied flow problems such as Fourier and Couette flows, normal shock wave, pressure-driven and thermally-driven channel flows and thermal diffusion in gas mixtures. The computational solver will bridge the gap between existing continuum and atomistic simulations and would enable scientists and engineers to address so far intractable non-equilibrium transport problems for low-speed gas mixture flows of fundamental and practical interest. The specific applications that will be addressed in this project include trace gas separation and gas analytical technologies as well as ultra-high-heat flux evaporation cooling for high-performance integrated circuits. The computation solver will be released to the research community, and the results will be integrated into courses to educate students. The new computational framework is based on the Discontinuous Galerkin Fast Spectral (DGFS) method which allows accurate deterministic solution of the full Boltzmann equation for arbitrary geometries and gas mixtures. The deterministic solution of the integro-differential Boltzmann equation is high order accurate in both physical and velocity space and time, free from statistical noise and sampling errors, and is particularly suitable for unsteady and low speed flow simulations. Due to the multi-dimensionality of physical and velocity phase space of the Boltzmann equation, the deterministic solution of rarefied flows is computationally demanding; hence, it requires solvers that are efficient on massively parallel architectures. The high-order h/p methods for the Boltzmann equation exhibit excellent parallel-scaling and serve as the basis of the proposed DEEKSHA framework. To broaden the impact of project, the computational solver will be released under a general, public license. The planned activities for dissemination to end-user communities include: a) workshops for developers and simulation bootcamps at conferences; b) integration of research results into courses taught at Purdue School of Astronautics and Aeronautics and Department of Mathematics as well as a webinar and interactive tool on Nanohub; and c) research experiences for undergraduate students through Purdue Engineering Summer Undergraduate Research Fellow program. "Deeksha" is a Sanskrit word which "loosely" means “where the mind of two entities become one, and they work towards a common goal”. I cowrote this proposal at 22, based on my Masters research work on "Discontinuous Galerkin Fast Spectral (DGFS)", which got funded for ~0.35 million ... Cheers!
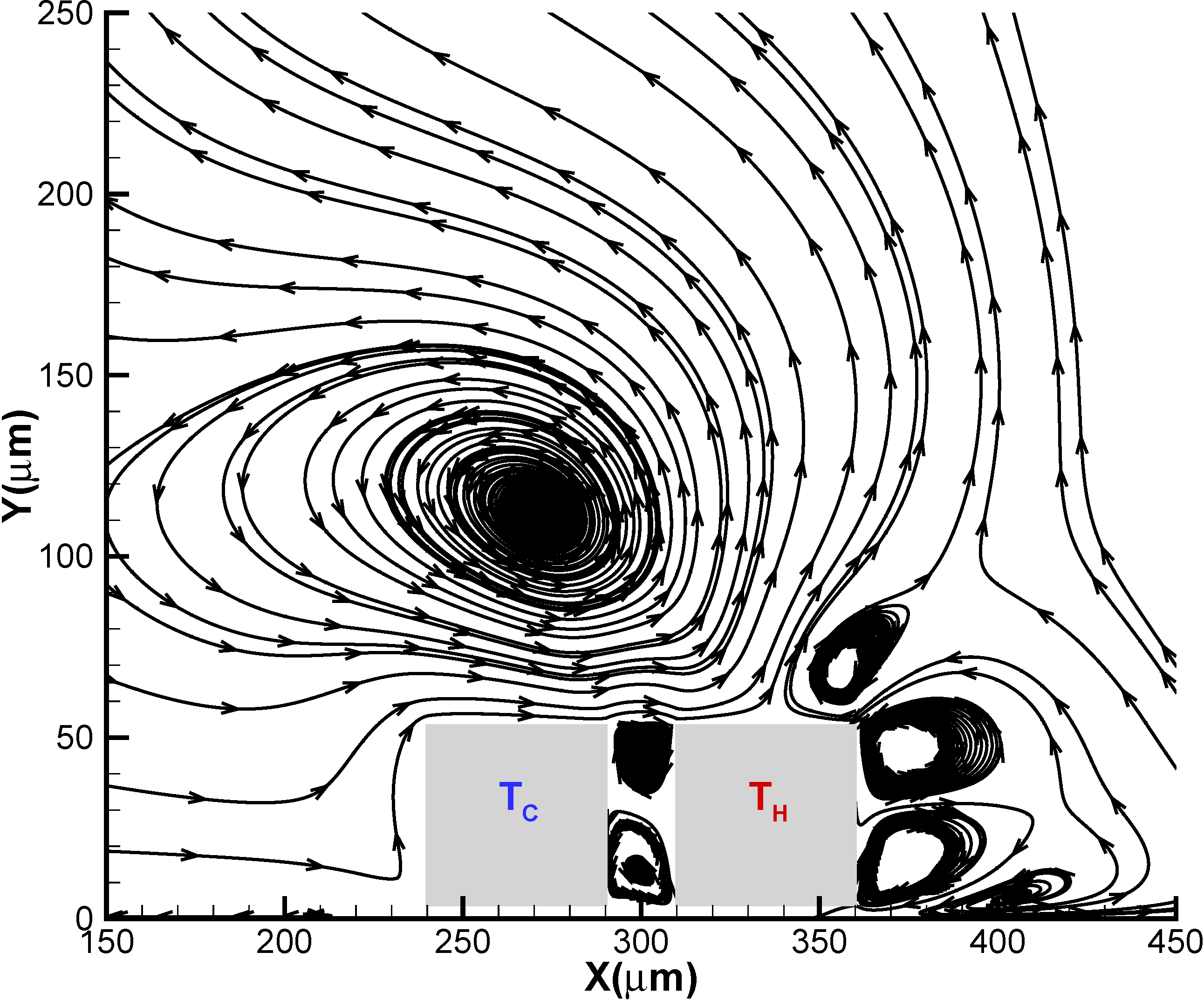
Quantification of thermally-driven flows in microsystems using Boltzmann equation in deterministic and stochastic contexts
Shashank Jaiswal, Aaron Pikus, Andrew Strongrich, Israel B. Sebastião, Jingwei Hu, and Alina A. Alexeenko
2019 American Institute of Physics (AIP), Physics of Fluids, Submitted: 30th April 2019, Accepted: 10th July 2019
Abstract: When the flow is sufficiently rarefied, a temperature gradient, for example, between two walls separated by a few mean free paths, induces a gas flow---an observation attributed to the thermo-stress convection effects at microscale. The dynamics of the overall thermo-stress convection process is governed by the Boltzmann equation---an integro-differential equation describing the evolution of the molecular distribution function in six-dimensional phase space---which models dilute gas behavior at the molecular level to accurately describe a wide range of flow phenomena. Approaches for solving the full Boltzmann equation with general inter-molecular interactions rely on two perspectives: one stochastic in nature often delegated to the direct simulation Monte Carlo (DSMC) method; and the others deterministic by virtue. Among the deterministic approaches, the discontinuous Galerkin fast spectral (DGFS) method has been recently introduced for solving the full Boltzmann equation with general collision kernels, including the variable hard/soft sphere models---necessary for simulating flows involving diffusive transport. In this work, the deterministic DGFS method; Bhatnagar-Gross-Krook (BGK), Ellipsoidal statistical BGK, and Shakhov kinetic models; and the widely-used stochastic DSMC method, are utilized to assess the thermo-stress convection process in MIKRA---Micro In-Plane Knudsen Radiometric Actuator---a microscale compact low-power pressure sensor utilizing the Knudsen forces. BGK model under-predicts the heat-flux, shear-stress, and flow speed; S-model over-predicts; whereas ESBGK comes close to the DSMC results. On the other hand, both the statistical/DSMC and deterministic/DGFS methods, segregated in perspectives, yet, yield inextricable results, bespeaking the ingenuity of Graeme Bird who laid down the foundation of practical rarefied gas dynamics for microsystems.
*Special issue dedicated to Graeme Bird
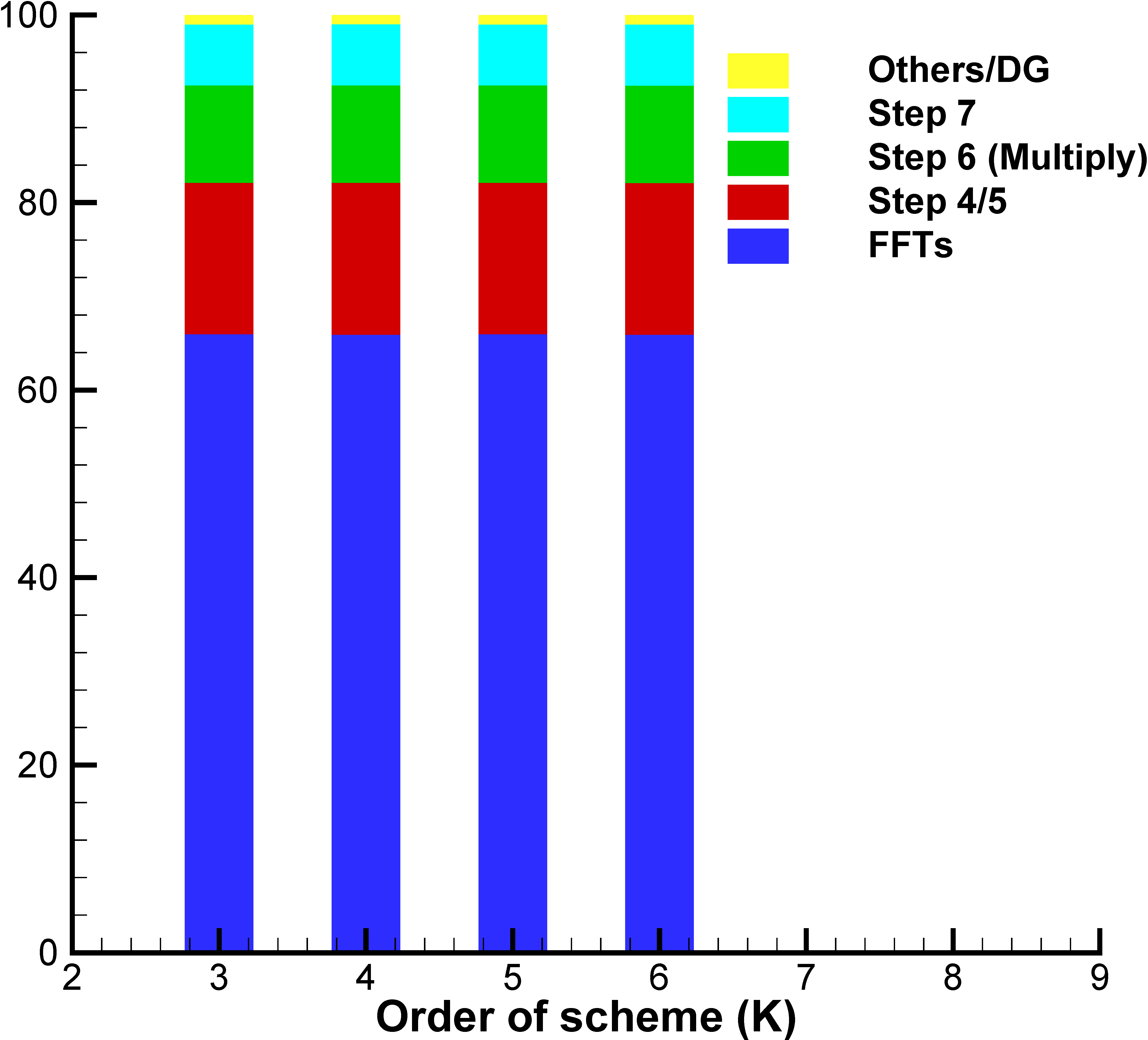
A discontinuous Galerkin fast spectral method for multi-species full Boltzmann on streaming multi-processors*
Shashank Jaiswal, Jingwei Hu, Julien K. Brillon, and Alina A. Alexeenko
2019 Association for Computing Machinery (ACM), Platform for Advanced Scientific Computing (PASC)
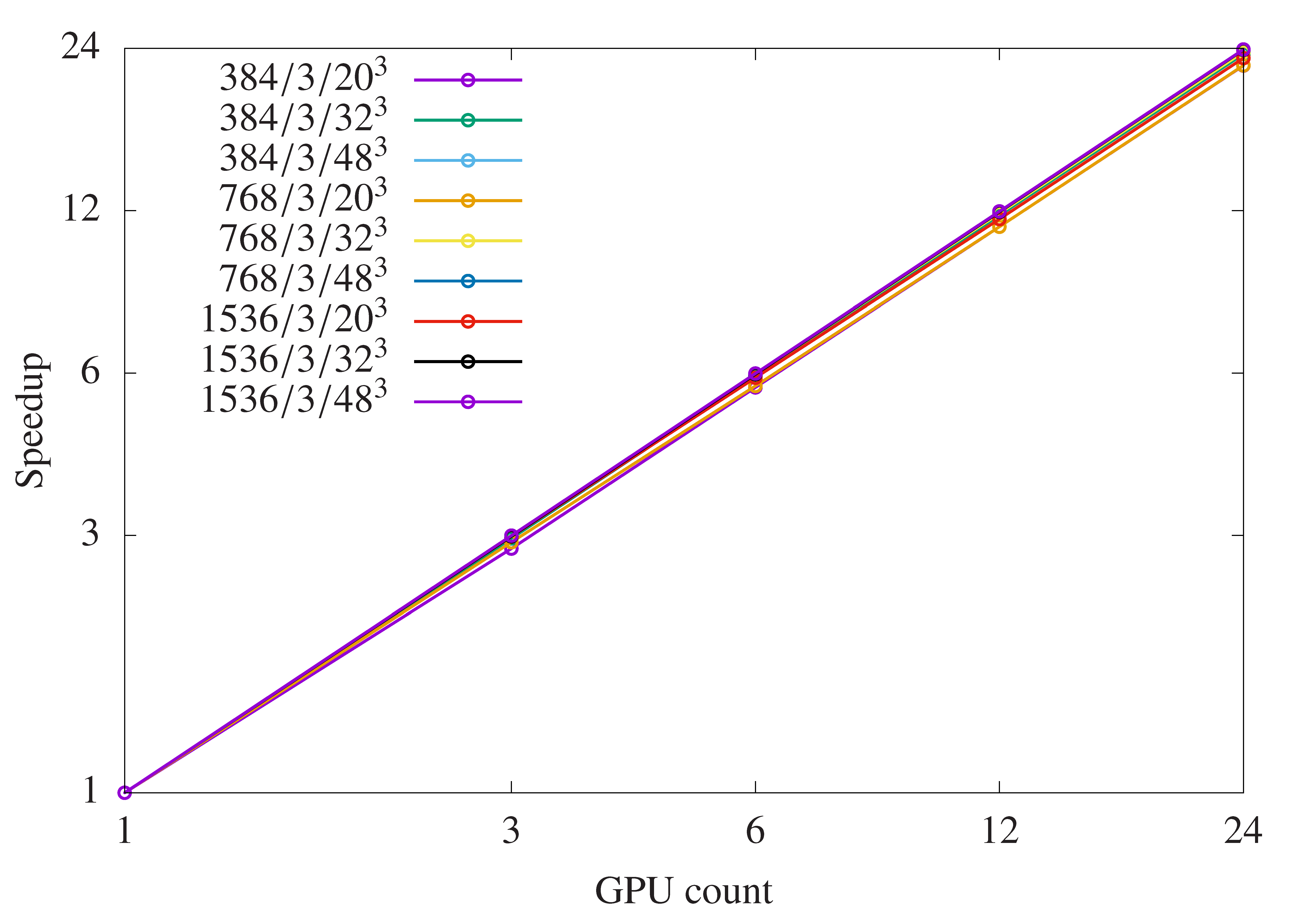
Abstract: When the molecules of a gaseous system are far apart, say in microscale gas flows where the surface to volume ratio is high and hence the surface forces dominant, the molecule-surface interactions lead to the formation of a local thermodynamically non-equilibrium region extending few mean free paths from the surface. The dynamics of such systems is accurately described by Boltzmann equation. However, the multi-dimensional nature of Boltzmann equation presents a huge computational challenge. With the recent mathematical developments and the advent of petascale, the dynamics of full Boltzmann equation is now tractable. We present an implementation of the recently introduced multi-species discontinuous Galerkin fast spectral (DGFS) method for solving full Boltzmann on streaming multi-processors. The present implementation solves the inhomogeneous Boltzmann equation in span of few minutes, making it at least two order-of-magnitude faster than the present state-of-art stochastic method---direct simulation Monte Carlo---widely used for solving Boltzmann equation. Various performance metrics, such as weak/strong scaling have been presented. A parallel efficiency of 0.96--0.99 is demonstrated on 36 Nvidia Tesla-P100 GPUs.
Solves the time-accurate full multi-species 1-D Couette flow in 138 seconds on a single GPU! The single species — not included in the paper, but available in the source/code repository — solves the time-accurate full 1-D Couette/Fourier/Oscillatory-Couette flow in ~6 seconds on a single GPU!
*Awarded ACM SIGHPC travel grant (1 out of total 4 awardees across the globe; 1 out of total 2 awardees from outside Europe)
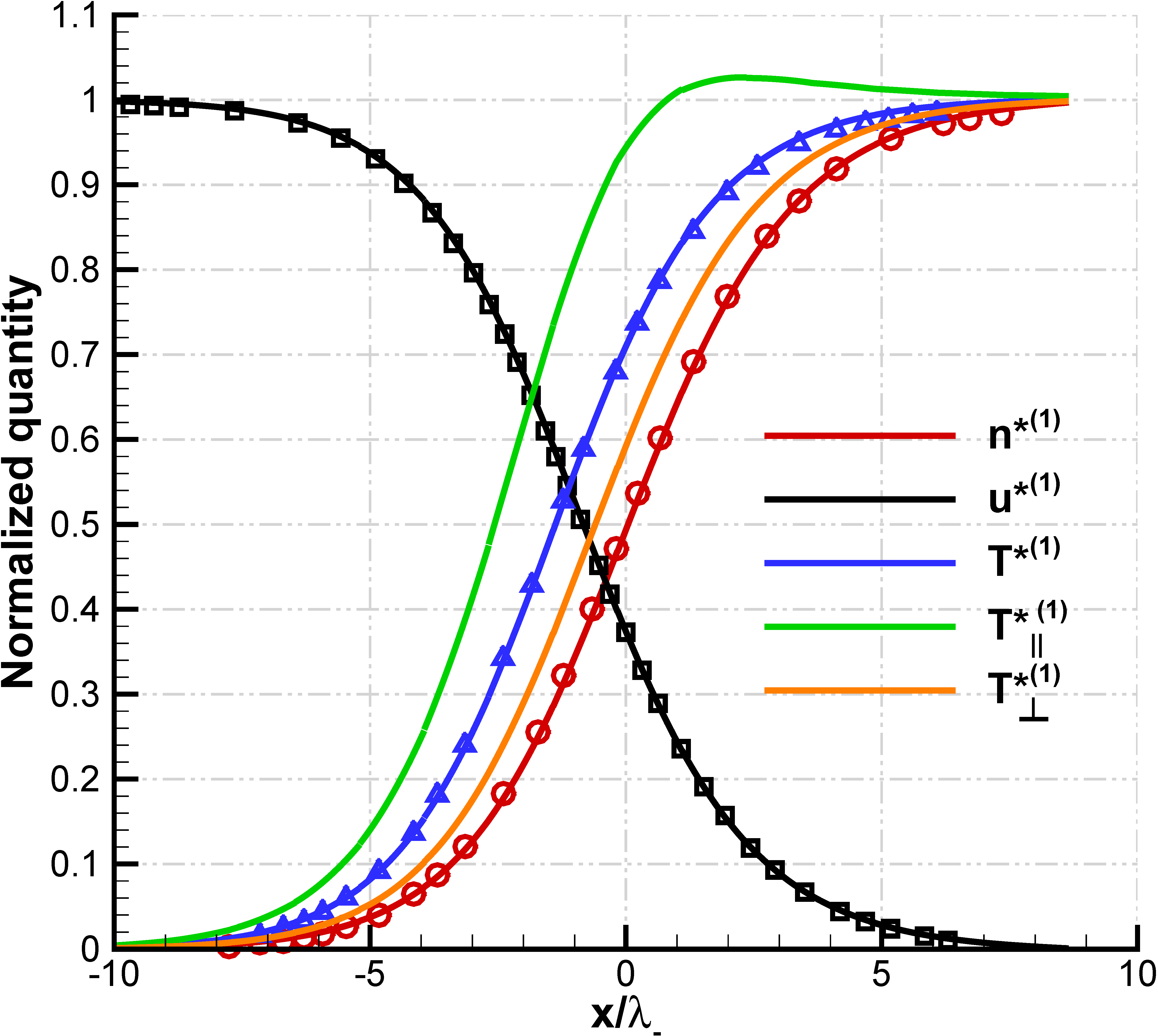
A discontinuous Galerkin fast spectral method for the multi-species full Boltzmann equation
Shashank Jaiswal, Alina A. Alexeenko, and Jingwei Hu
2019 Elsevier, Computer Methods in Applied Mechanics and Engineering, Submitted: 14th Dec 2018, Accepted: 9th April 2019
Abstract: We introduce a fast Fourier spectral method for the multi-species Boltzmann collision operator. The method retains the riveting properties of the single-species fast spectral method (Gamba et al. SIAM J. Sci. Comput., 39 pp. B658--B674 2017) including: (a) spectral accuracy, (b) reduced computational complexity compared to direct spectral method, (c) reduced memory requirement in the precomputation, and (d) applicability to general collision kernels. The fast collision algorithm is then coupled with discontinuous Galerkin discretization in the physical space (Jaiswal et al. J. Comp. Phys., 378 pp. 178--208 2019) to result in a highly accurate deterministic method (DGFS) for the full Boltzmann equation of gas mixtures. A series of numerical tests is performed to illustrate the efficiency and accuracy of the proposed method. Various benchmarks highlighting different collision kernels, different mass ratios, momentum transfer, heat transfer, and in particular the diffusive transport have been studied. The results are directly compared with the direct simulation Monte Carlo (DSMC) method.
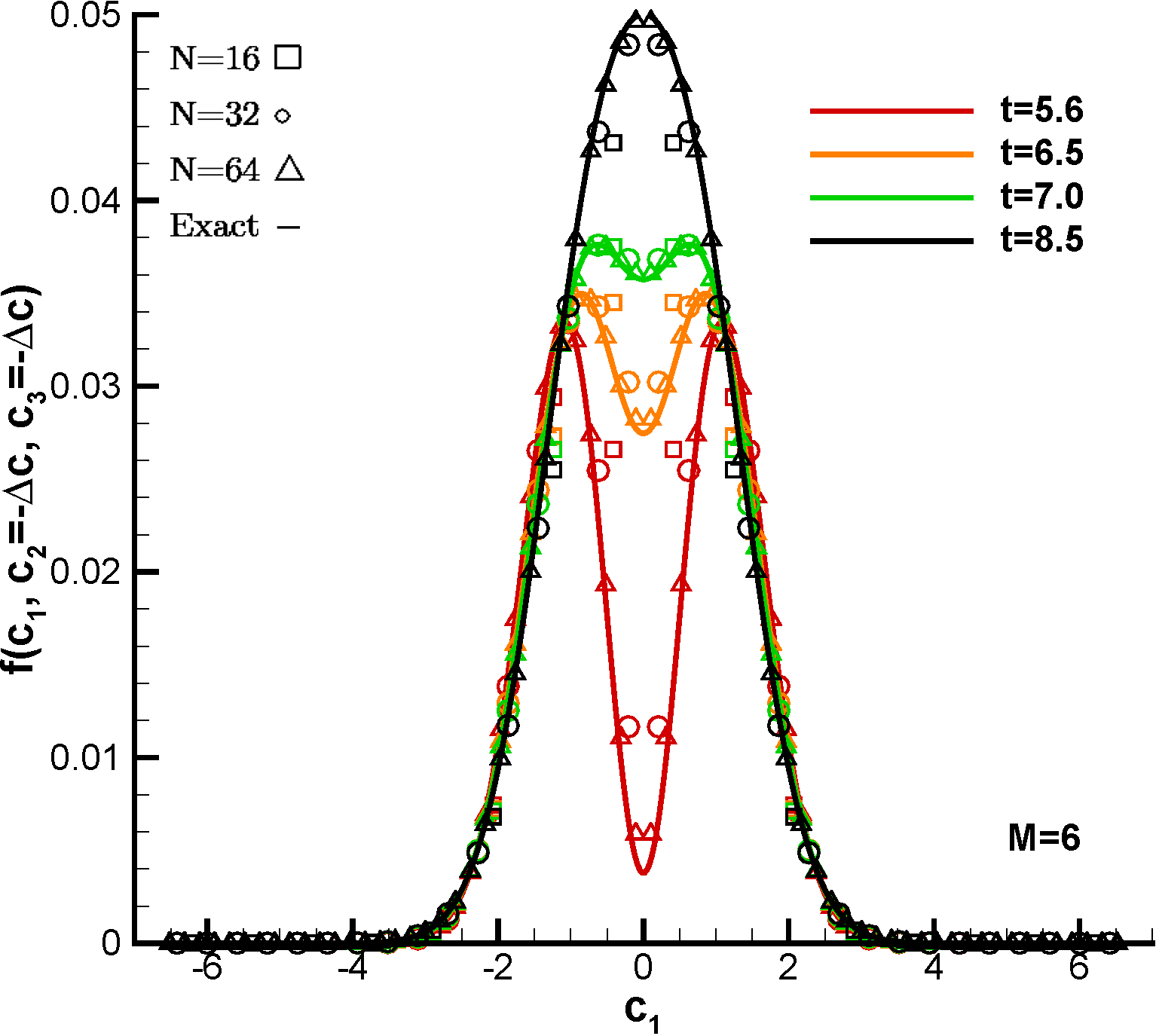
A discontinuous Galerkin fast spectral method for the full Boltzmann equation with general collision kernels
Shashank Jaiswal, Alina A. Alexeenko, and Jingwei Hu
2018 Elsevier, Journal of Computational Physics, Submitted: 29th March 2018, Accepted: 1st November 2019
Abstract: The Boltzmann equation, an integro-differential equation for the molecular distribution function in the physical and velocity phase space, governs the fluid flow behavior at a wide range of physical conditions, including compressible, turbulent, as well as flows involving further physics such as non-equilibrium internal energy exchange and chemical reactions. Despite its wide applicability, deterministic solution of the Boltzmann equation presents a huge computational challenge, and often the collision operator is simplified for practical reasons. In this work, we introduce a highly accurate deterministic method for the full Boltzmann equation which couples the Runge-Kutta discontinuous Galerkin (RKDG) discretization in time and physical space (Su et al., Comp. Fluids, 109 pp. 123-136, 2015) and the recently developed fast Fourier spectral method in velocity space (Gamba et al., SIAM J. Sci. Comput., 39 pp. B658–B674, 2017). The novelty of this approach encompasses three aspects: first, the fast spectral method for the collision operator applies to general collision kernels with little or no practical limitations, and in order to adapt to the spatial discretization, we propose here a singular-value-decomposition based algorithm to further reduce the cost in evaluating the collision term; second, the DG formulation employed has arbitraryhigh order of accuracy at element-level, and has shown to be more efficient than the finite volume method; thirdly, the element-local compact nature of DG as well as our collision algorithm is amenable to effective parallelization on massively parallel architectures. The solver has been verified against analytical Bobylev-Krook-Wu solution. Further, the standard benchmark test cases of rarefied Fourier heat transfer, Couette flow, oscillatory Couette flow, normal shock wave, lid-driven cavity flow, and thermally driven cavity flow have been studied and their results are compared against direct simulation Monte Carlo (DSMC) solutions with equivalent molecular collision models or published deterministic solutions.
Fast Deterministic Solution of the Full Boltzmann equation on Graphics Processing Units
Shashank Jaiswal, Jingwei Hu, and Alina A. Alexeenko
2018 Americal Institute of Physics, 31st Rarefied Gas Dynamics Symposium
Abstract: The Boltzmann equation, a six-dimensional integro-differential equation, governs the fluid flow behavior at molecular level for a wide range of physical phenomena, including shocks, turbulence, diffusion, and non-equilibrium chemistry which are beyond the reach of continuum fluid flow modelling based on the Navier-Stokes equations. Despite Boltzmann equation's wide applicability, its deterministic solution presents a huge computational challenge, and has been so far tractable only in simplified forms. We present the `first' Discontinuous Galerkin Fast Spectral (DGFS) method (Jaiswal et al. J. Compt. Phys. 2018) for solving the full Boltzmann equation on streaming multi-processors. The proposed method is flexible and robust allowing: a) arbitrary unstructured geometries, b) control of spatial accuracy using high-order polynomial approximation without compromising simulation stability, c) exponential error convergence (spectral accuracy) in velocity space, and d) compact nature of DG as well as collision operator thus minimizing communication and maximizing parallel efficiency. The DG operators (for instance gradient, curl, etc), as well as the collision operator is applied in an element-local way, with flux-based element-to-element coupling. It is this locality that equips DGFS with strong parallel performance on streaming multi-processors. In the present work, we describe, devise and implement DGFS for General-Purpose Graphics Processing Units (GP-GPU). We consider the simulations of 0D spatially homogeneous, and rarefied 1D Fourier heat transfer. A speedup of approximately 10--100x, and parallel efficiency of 0.95 is demonstrated on multi-CPU/multi-GPU architectures. It is this speedup that now allows researchers to solve problems within a day that would otherwise take months on traditional CPUs. The key optimizations and techniques used to achieve these GPU performance results have been highlighted.

FEMTA Micropropulsion System Characterization by DSMC
Shashank Jaiswal, Israel B. Sebastião, Andrew Strongrich, and Alina A. Alexeenko
2018 Americal Institute of Physics, 31st Rarefied Gas Dynamics Symposium
Abstract: CubeSats with compact, low-power attitude-control systems open up opportunities for deep-space missions as well as cost-effective constellation-based satellites targeting remote sensing and communication applications. Film Evaporation MEMS Tunable Array (FEMTA) is a micropropulsion technology that employs thermally-controlled surface tension in a microscale capillary for vaporization of liquid water propellant. FEMTA thrusters generate tunable thrust on the order of micro-newton with a thrust-to-power ratio of about 230 $\mu N/W$. Because of the small scale capillary on the order of 10 micron and below, the rarefied flow effects are significant for the vapor flow in this system. In the present work, the direct simulation Monte Carlo (DSMC) SPARTA solver is used to numerically evaluate two key aspects of the FEMTA system: a) the evaporation of the propellant in the quiescent system-state, i.e., without heaters actuation, and b) effect of plume expansion on the CubeSat walls to inform thrust cell placement. Both factors affect the propellant consumption and system lifetime.
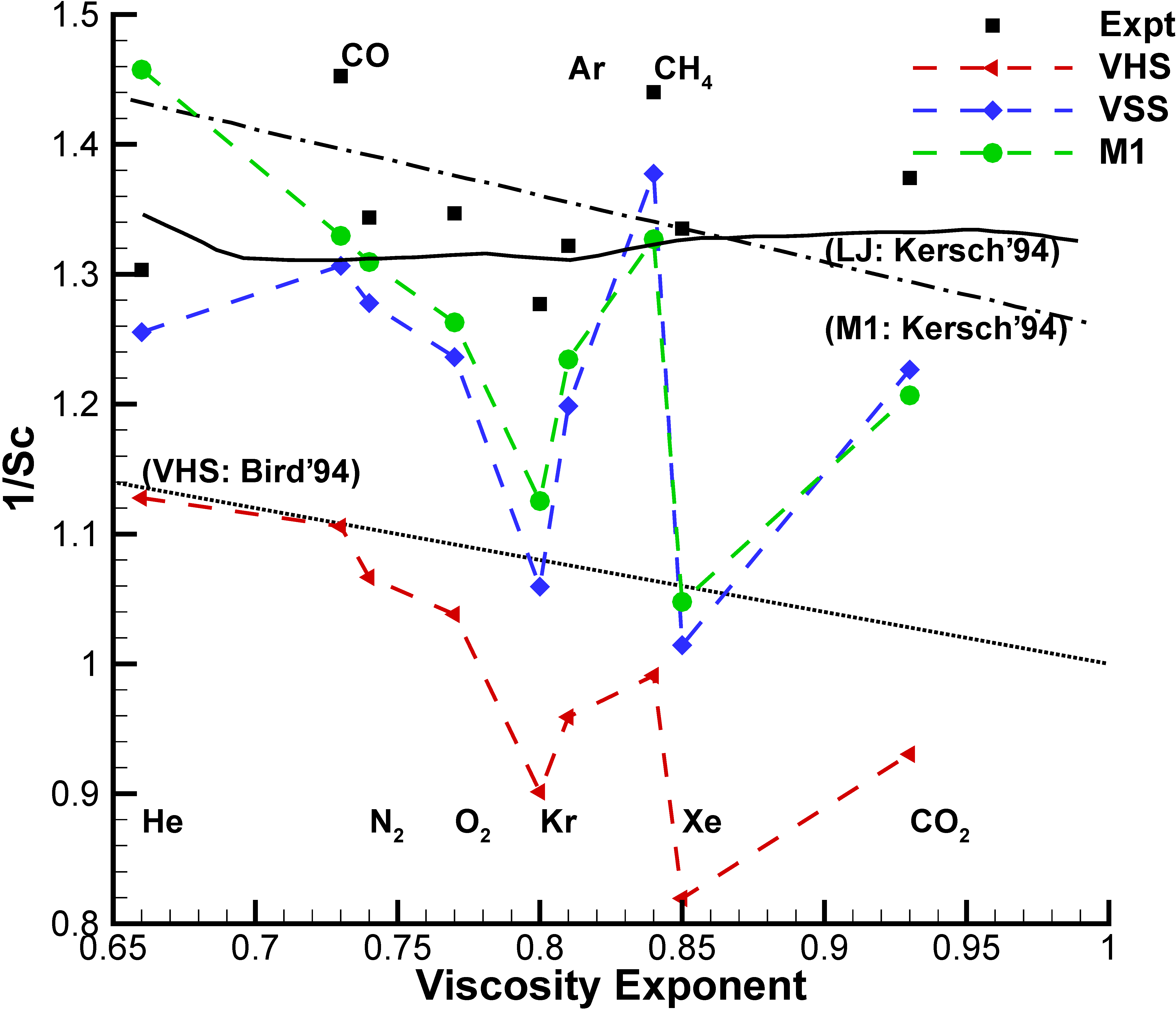
DSMC-SPARTA Implementation of M-1 Scattering Model
Shashank Jaiswal, Israel B. Sebastião, and Alina A. Alexeenko
2018 Americal Institute of Physics, 31st Rarefied Gas Dynamics Symposium
Abstract: The direct simulation Monte Carlo (DSMC) method takes advantage of phenomenological models that can reproduce macroscopic transport and chemistry rates from elementary collisional kinetic data. As in classical kinetic theory of gases, reproduction of viscosity, thermal conductivity, and diffusion coefficients rely on the scattering dynamics that particles undergo during collisions. In this work, the still under-explored Kersch & Morokoff's (M-1) phenomenological scattering model is implemented in the DSMC-SPARTA solver. In essence, this model is a modification of the well-known variable hard/soft sphere (VHS/VSS) models for repulsive interactions to have a linear distribution of scattering angles in terms of the impact parameter. Such a feature, in general, better represents observations from quasi-classical trajectory (QCT) calculations based on ab-initio data. While the energy-dependence of M-1 collision cross-section remains the same as in VHS model, M-1 predicts more realistic diffusion coefficients that are ~20% higher than those obtained with VHS scattering law. M1-model recovers Schmidt numbers (Sc) within 5% of those obtained from the Lennard-Jones (LJ) and Abrahamson (Abr) interaction models at temperatures as high as 11,000 K. A distinct feature of M-1 model is the absence of the VSS anisotropic scattering fitting parameter ($\alpha$) and its applicability for large range of temperatures. In this aspect, without additional computational costs, M-1 model is potentially more suitable for conditions in which experimental diffusion-data is not available to fit the VSS parameter over a large temperature interval.
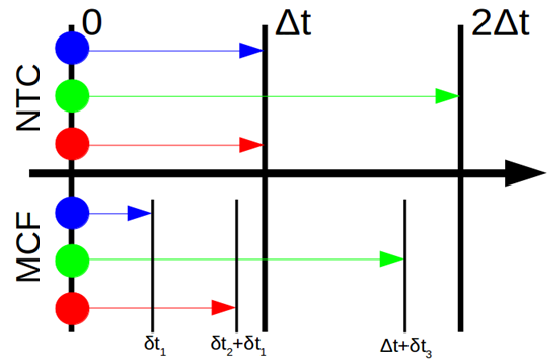
DSMC-SPARTA Implementation of Majorant Collision Frequency Scheme
Aaron Pikus, Israel B. Sebastião, Shashank Jaiswal, Michael Gallis, and Alina A. Alexeenko
2018 Americal Institute of Physics, 31st Rarefied Gas Dynamics Symposium
Abstract: The direct simulation Monte Carlo (DSMC) method takes a stochastic approach to solving the Boltzmann equation, and is commonly used to model rarefied gas flows. The simulation domain is split into cells, in which computational representative particles mimic the real flow. Since millions to billions of particles need to be tracked, these models need to be both accurate and computationally efficient. Even with high-fidelity collision models, the overall accuracy of the DSMC outcome strongly depends on its ability to predict the correct number of collision events per simulated time step, i.e. the collision frequency. The two most popular approaches for this purpose are the no-time-counter (NTC) and majorant collision frequency (MCF) schemes. While the NTC scheme is designed to reproduce the average collision time for a sufficiently large sample sizes, the MCF scheme has the advantage of reproducing the exact Poisson distribution of collision time as well as the mean collision time with a reduced sample size. Both schemes have linear complexity of O(N), with N being the number of particles. In this work, we implement the MCF scheme in SPARTA, an open source DSMC solver. A series of numerical tests are performed to illustrate the efficiency and accuracy of both the schemes. Various benchmarks highlighting unsteady, compression, and expansion problems are studied.
Undergraduate:
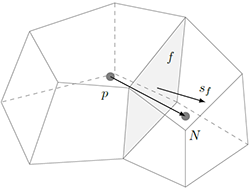
An Efficient GPU Parallelization for Arbitrary Collocated Polyhedral Finite Volume Grids and Its Application to Incompressible Fluid Flows
Shashank Jaiswal, Rajesh Reddy, Raja Banerjee , Shingo Sato, Daisuke Komagata, Makoto Ando, and Jun Okada
2016 IEEE, 23rd International Conference on High Performance Computing Workshops (HiPCW)
Abstract: This paper presents GPU parallelization for a computational fluid dynamics solver which works on a mesh consisting of polyhedral cells, where each cell has an arbitrary number of faces and each face has an arbitrary number of vertices. The parallelization is achieved using NVIDIAs compute unified device architecture (CUDA). The developed code specifically targets performance improvement on NVIDIA Tesla accelerator GPUs. The implementation has been carried out in a general purpose open-source CFD framework namely OpenFOAM which is capable of solving arbitrary flow problems involving complex geometries with polyhedral unstructured grids. The present work considers incompressible flow simulations, where solving pressure Poisson equation is the most computationally expensive step. The Poisson equation is solved using conjugate gradient method preconditioned by algebraic multigrid method. This part of the solver is outsourced by OpenFOAM to GPU. The GPU pressure Poisson solver acceleration is determined with respect to OpenFOAM serial version (single cpu core) and MPI parallelized version (8 cpu cores). The GPU solver acceleration was tested by simulating a standard benchmark test case called lid driven cavity flow for different grid sizes. The current GPU based solver has shown a speedup of approximately 16x when compared to single cpu core and 3.3x when compared to OpenFOAM MPI version using 8 cpu cores, for a grid size of 5 million cells.
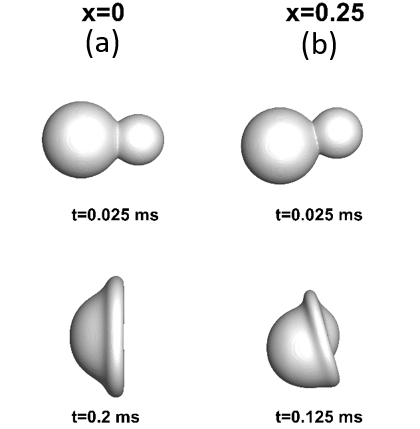
High fidelity simulations of binary collisions of liquid drops
Sarang Holay, Rajesh Reddy, Shashank Jaiswal, and Raja Banerjee
2016 ILASS-Asia, 18th Annual Conference on Liquid Atomization and Spray Systems
Abstract: The phenomena of binary droplet collision is seen in dispersed phase systems such as sprays in internal combustion engines, gas turbines, etc. The present work aims at understanding off-center collision dynamics of two droplets of unequal sizes. High fidelity simulations are performed using three dimensional, two phase- finite volume based solver in OpenFOAM platform. Volume of fluid (VOF) method is used for interface capturing. Navier-Stokes equations are solved using a projection algorithm. Simulations are done with MPI parallelization to meet the computational demand. The solver is validated against droplet splashing benchmark test case. The present numerical results study the effect of impact parameter and diameter ratio on droplet collision dynamics.
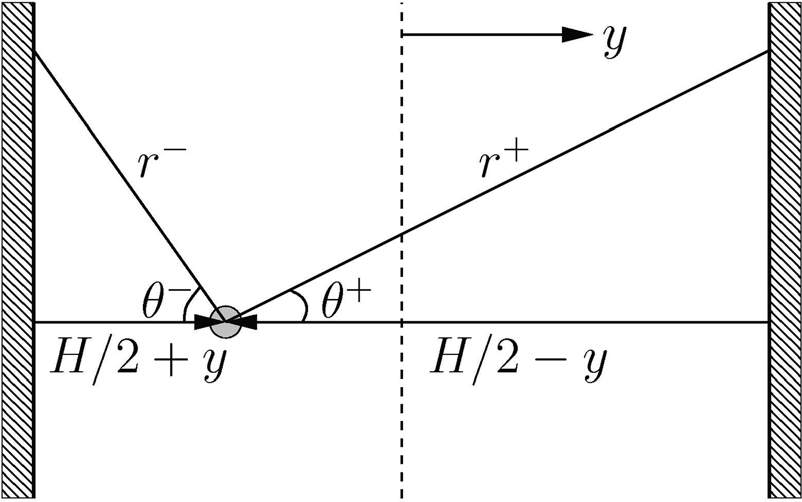
Implementation of Knudsen Layer Effects In Open Source CFD Solver for Effective Modeling of Microscale Gas Flows
Shashank Jaiswal, and Nishanth Dongari
2015 ISHMT-ASTFE, 1st International and 23rd National Heat and Mass Transfer Conference, Liquid Propulsion Systems Center (ISRO), Thiruvananthapuram, India